Being an engineer, excellent comprehension is necessary on how to make structural analysis for buildings, bridges, and other structures.
Structural analysis is the calculations of the magnitudes of forces, stresses, strains and deflections or deformations of structures when LOADS, external forces are being applied and exerted on structures.
 |
| Subjects and Necessary Interest |
The readers of this blogpost who are not engineers may very well amaze and ask; "Where in the world did they get these Loads?" "What on earth do they think they are weighing?" That very crucial and logical questions will be answered in this blogpost.
1. Specifications, Building Codes, and Bridge codes.
Designers must look for appropriate Specification and Codes. National and Local government have published building codes, bridge and highway codes for the safety purposes of the public, which control the construction of different types of structures within their country. Actually, these codes are laws or ordinances that specify design loads, design stresses, construction types, material quality among others. Not many specifications published recommended practices for local and national use. These codes and specifications are not enforceable legally, nevertheless, unless it is embodied in their national building code, and made integral part of a particular contract of projects. Among these organizations are;
- ASCE -American Society of Civil Engineers
- AASHTO -American Association of State Highway and Transportation official
- AISC -American Institute of Steel Construction
- ACI -American Concrete Institute
- ASEP -Association of Structural Engineers of the Philippines
The following specifications published by the above-mentioned organizations oftenly are used to estimate the maximum load and minimum loads to which the bridges, buildings, and other structures may be subjected during their estimated lifetimes.
- Minimum Design Loads for Buildings and other Structures, published by ASCE 7-2005 edition;
- AASHTO LRFD Bridge Design Specifications, published by AASHTO;
- Specifications for Structural Steel Buildings- 2010, published by AISC;
- Steel Construction Manual, 14 edition, published by AISC;
- National Structural Code of the Philippines, volume 1 -Buildings, volume 2 -Bridges, published by ASEP.
Readers of this bolgpost should pay attention that reasonable and clearly written codes are really helpful to designers.
The great pyramid in Egypt, the Parthenon in Athens, and the great Roman bridges and aqueducts built by ANCIENT BUILDERS were controlled by few specifications, which precisely is true. It should be spoken that only few number of these great structures were built over many 100 of years or centuries, and were ostensibly built WITHOUT CONSIDERATION or CARE about COST OF LABOR, MATERIAL, OR HUMAN LIFE. The were built probably by intuitions, and certain RULES OF THUMBS ("SINUBOK LAMANG" at KAWALAN O walang RASYONAL na PROSESO -in local dialect), developed by seeing the minimum size or strength of members that would fail only under certain given conditions. Their NUMEROUS FAILURES are NOT RECORDED in HISTORY, only their SUCCESSES ENDURED.
For the information and guidance of all readers of this blogpost, notably the ordinary engineers in the Philippines, I would like to give emphasis to them, that the national government agencies in the Philippines (DPWH, NIA, DOTC, DSWD-Kalahi) had adopted the latest international recommended practices and codes, like the ASCE standards, ACI Codes, AREA Code, AISC standards, ASTM standards. In view of the fact that ENGINEERING EDUCATION in the Philippines is AMERICAN ORIENTED, the ASEP committee decided to recommend the adoption of the Earthquake Regulation as provided in the Uniform Building Code.
Hence, the Association of Structural Engineers of the Philippines (ASEP) published National Structural Code of the Philippines as a referral code of the National Building Code of the Philippines. The NSCP code reflects the continuing technical advances in structural engineering and the latest seismic design practice for earthquake resistant structures, viz:
- Reinforced concrete design conforms to the provisions of the American Concrete Institute (ACI-318) Code.
- Bridges and highways specifications are patterned after the provisions of the AASHTO.
- The ASEP recommended Earthquake Regulations are patterned after the provisions of the Uniform Building Code (SEAOC) of the United States of America.
- The Minimum Design Loads for Buildings and other structures conforms to the provisions of American Society of Civil Engineers (ASCE 7-2005).
- Steel and Iron specifications are patterned after the provisions of the American Institute of Steel Construction (AISC) and American Standards for Testing of Materials (ASTM).
The Department of Public Works and Highways (DPWH) issued Department Order No.82-1, 1982;
Dead Loads: Weight of the structure under consideration, as well as any fixtures that are permanently attached to it.
Live Loads: They include occupancy loads, warehouse materials, construction loads, overhead service cranes, and equipment loads. They are gravity induced.
Environmental Loads: For Buildings, they are caused by rain, snow, wind, and earthquake.
2.1 Dead Loads
2.1.1 Weights of Common Building Materials
Reinforced Concrete -150 pcf
Concrete Hollow blocks (no plaster) -44 psf
G.I. roofing -2.5 psf
Suspended Ceiling -2 psf
Hardwood flooring -4 psf
2.2 Live Loads
2.2.1 Typical Uniformly Distributed Live Loads:
Residential dwelling areas -40 psf
Classrooms in schools -40 psf
Offices in office buildings -50 psf
Retail stores -first floor -100 psf
Retail stores -upper floor -75 psf
Dance hall and ballrooms -100 psf
Library reading rooms -60 psf
2.3. Lateral Loads:
There are certain loads that are almost always applied horizontally.
Wind Loads, soil pressures, hydrostatic pressures, forces due to earthquakes, centrifugal forces, and longitudinal forces.
2.3.1 Wind Loads
A.1 The basic reference equivalent static pressure in the critical local wind speed.
Formula:
QS = 2 ^ 0.0000483V
Where:
V = wind velocity in KPH
qs = in kPa
Applicable to Duchemin formula (developed in 1829 by French Army Officer, Col. Duchemin)
1. Duchemin Formula..
Pn = p (2 sinϴ/1 + sin^2ϴ) -- Wind Pressure normal to an inclined roof surface.
2. ASCE Recommendation..
2.3.2 Earthquakes Loads or Forces E
2.3.2.1 Static Lateral Force Procedure
Formulas:
A. Uniform Building Code
Where:
Z = Seismic Zone Coefficient
I =Importation factor
C =Coefficient depending on the Soil condition and the period of the structure
Rw =response modification factor which represents the ductility of the structural system
W =Weight of the structure or seismic dead load
B. ASCE and IBC Code (International Building Code)
3. SYSTEM LOADING
3.1 Tributary Area
3.2 LOADING CONDITIONS for STRENGTH DESIGN
3.2.1 Load Combinations
A. ACI 318 Code -1989 up to 1995
U = 1.4D + 1.7L ---------------------------- 9.2.1
U = 0.75 [ 1.4D +1.7 L (+ -) 1.7WL] ------- 9.2.2
U = 0.9D (+-) 1.3WL
U = 0.75 [ 1.4D + 1.7L (+ -) 1.7 * 1.1E ] ---- 9.2.3
U = 0.9D (+-) 1.3*1.1E
B. ACI 318-2002 Code
U = 1.2D + 1.6L ---------------------------------9-2
U = 1.2D + 1.6W + 1.0L + 0.5(Lr or S or R) --- 9-4
U = 1.2D + 1.0E +1.0L + 0.2S ----------------- 9-5
U = 0.9D + 1.6W + 1.6H ------------------------9-6
U = 0.9D (+ -) 1.0E + 1.6H --------------------- 9-7
C. ASCE 7-95 and ASCE 7-05 Recommended Load Combinations for Building Structures and adopted in the ACI 318-2002.
LRFD = 1.2D + 1.6L
LRFD = 1.2D + 1.6W + (0.5 or 1.0)*L + 0.5(Lr or S or R)
LRFD = 1.2D + 1.0E + (0.5 or 1.0)*L + 0.2S
LRFD = 0.9D + 1.6W + 1.6H
LRFD = 0.90D + 1.0E +1.6H
3.3 PLACING LOADS on the STRUCTURES
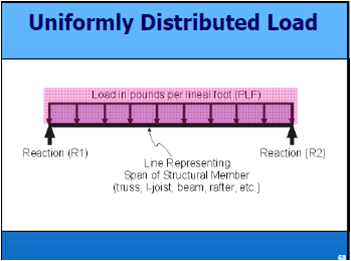
3.3.1 Uniformly Distributed Loaded
3.3.2 Point Loads
4. REACTIONS, SHEAR and MOMENT DIAGRAMS
The most important phase in Structural Engineering is the knowledge of Reactions and understanding of Shear and Moment diagrams and their formations, and/or the FBD diagram sketches.
Simple Beam with Overhang at one support - uniformly distributed load:
5. TWO and THREE DIMENSIONAL TRUSSES (this subject will not be discussed in this blogpost)
5.1 Types of Trusses
7. STATICALLY INDETERMINATE STRUCTURES
7.1 Classical Method of Analysis:
These methods are basically of HISTORICAL interest and are almost never used in practice.
7.2 Modern Method of Analysis
A. Approximate Method:
B. EXACT METHOD OF ANALYSIS:
8. STRESSES Calculations: (NSCP 1.4.2, ACI Code 8.3)
8.1 Bending Moments (Beams, Columns):
8.2 Shear Forces:
8.3 Axial Forces:
8.4 Torsion Forces
References:
"For the guidance and compliance of all concerned and pursuant to section 203 of PD 1096, the National Structural Code for Buildings a referral code of the NBC (PD 1096) to reflect the following;
- In Chapter 2, lateral forces, are revised to reflect the provisions of the Uniform Building Code (UBC-SEAOC)
- Chapter 4, Steel and Iron, conforms to the provisions of the American Institute of Steel Construction (AISC).
- Chapter 5, Concrete, conforms to American Concrete Institute -ACI 318 Code with the equations in SI Units."
Dead Loads: Weight of the structure under consideration, as well as any fixtures that are permanently attached to it.
Live Loads: They include occupancy loads, warehouse materials, construction loads, overhead service cranes, and equipment loads. They are gravity induced.
Environmental Loads: For Buildings, they are caused by rain, snow, wind, and earthquake.
2.1 Dead Loads
2.1.1 Weights of Common Building Materials
Reinforced Concrete -150 pcf
Concrete Hollow blocks (no plaster) -44 psf
G.I. roofing -2.5 psf
Suspended Ceiling -2 psf
Hardwood flooring -4 psf
 |
| Minimum Densities for Design Loads from materials (Source: ASCE 7 Standard) |
2.2 Live Loads
 |
| Floor Live Load |
2.2.1 Typical Uniformly Distributed Live Loads:
Residential dwelling areas -40 psf
Classrooms in schools -40 psf
Offices in office buildings -50 psf
Retail stores -first floor -100 psf
Retail stores -upper floor -75 psf
Dance hall and ballrooms -100 psf
Library reading rooms -60 psf
 |
| Minimum Uniformly Distributed Live Loads (Source: ASCE 7 Standard) |
 |
| Minimum Uniformly Distributed Live Loads (Source: ASCE 7 Standard) |
2.3. Lateral Loads:
There are certain loads that are almost always applied horizontally.
Wind Loads, soil pressures, hydrostatic pressures, forces due to earthquakes, centrifugal forces, and longitudinal forces.
2.3.1 Wind Loads
A.1 The basic reference equivalent static pressure in the critical local wind speed.
Formula:
QS = 2 ^ 0.0000483V
Where:
V = wind velocity in KPH
qs = in kPa
Applicable to Duchemin formula (developed in 1829 by French Army Officer, Col. Duchemin)
1. Duchemin Formula..
Pn = p (2 sinϴ/1 + sin^2ϴ) -- Wind Pressure normal to an inclined roof surface.
2. ASCE Recommendation..
 |
| ASCE 7-05 Wind Pressures Formula |
 |
| Wind External and Internal Pressures |
 |
| Internal Wind Pressures |
2.3.2 Earthquakes Loads or Forces E
2.3.2.1 Static Lateral Force Procedure
Formulas:
A. Uniform Building Code
 |
| 1988 -1994 UBC Formula for Base Shear |
Z = Seismic Zone Coefficient
I =Importation factor
C =Coefficient depending on the Soil condition and the period of the structure
Rw =response modification factor which represents the ductility of the structural system
W =Weight of the structure or seismic dead load
 |
| 1997 UBC Formula for Base Shear |
B. ASCE and IBC Code (International Building Code)
 |
| ASCE 7-2005 Base Shear, V Formula |
 |
| ASCE 7-05 and IBC 2006 Seismic Base Shear and Horizontal Forces |
3. SYSTEM LOADING
3.1 Tributary Area
.png) |
| Column Tributary |
.png) |
| Girder Tributary Area |
3.2 LOADING CONDITIONS for STRENGTH DESIGN
3.2.1 Load Combinations
A. ACI 318 Code -1989 up to 1995
U = 1.4D + 1.7L ---------------------------- 9.2.1
U = 0.75 [ 1.4D +1.7 L (+ -) 1.7WL] ------- 9.2.2
U = 0.9D (+-) 1.3WL
U = 0.75 [ 1.4D + 1.7L (+ -) 1.7 * 1.1E ] ---- 9.2.3
U = 0.9D (+-) 1.3*1.1E
B. ACI 318-2002 Code
U = 1.2D + 1.6L ---------------------------------9-2
U = 1.2D + 1.6W + 1.0L + 0.5(Lr or S or R) --- 9-4
U = 1.2D + 1.0E +1.0L + 0.2S ----------------- 9-5
U = 0.9D + 1.6W + 1.6H ------------------------9-6
U = 0.9D (+ -) 1.0E + 1.6H --------------------- 9-7
C. ASCE 7-95 and ASCE 7-05 Recommended Load Combinations for Building Structures and adopted in the ACI 318-2002.
LRFD = 1.2D + 1.6L
LRFD = 1.2D + 1.6W + (0.5 or 1.0)*L + 0.5(Lr or S or R)
LRFD = 1.2D + 1.0E + (0.5 or 1.0)*L + 0.2S
LRFD = 0.9D + 1.6W + 1.6H
LRFD = 0.90D + 1.0E +1.6H
3.3 PLACING LOADS on the STRUCTURES
3.3.1 Uniformly Distributed Loaded
3.3.2 Point Loads
 |
| Simple Beam with Single Point Load |
 |
| Simple Beam with Multiple Point Loads |
4. REACTIONS, SHEAR and MOMENT DIAGRAMS
The most important phase in Structural Engineering is the knowledge of Reactions and understanding of Shear and Moment diagrams and their formations, and/or the FBD diagram sketches.
 |
| Reaction equation for Simple Beam with Uniform Load |
 |
| Reaction for Simple Beam with Triangular Load |
Simple Beam with Overhang at one support - uniformly distributed load:
 |
| Beam overhanging at one support, Shear and Moment diagram, V, M -Equations |
5. TWO and THREE DIMENSIONAL TRUSSES (this subject will not be discussed in this blogpost)
5.1 Types of Trusses
- Howe Truss (William Howe -patented in 1840, iron truss was introduced in 1844, Jacoby-p10)
- Warren Truss (Originated in England about 1840)
- Whipple Truss (Squire Whipple -1847)
- Fink Truss (introduced by American Albert Fink, Jacoby1 -p170)
- Pratt Truss -(patented in 1844, Jacoby1-p155)
- Bollman Truss - (Wendall Bollman-patented in 1851, Jacoby1-p152)
- AASHTO - Bridges and Specifications, 1944 to 2005 standard and specifications
- NSCP Code -Volume 2 -Bridges.
 |
| Moving Loads (Truck Loading) Analysis |
7. STATICALLY INDETERMINATE STRUCTURES
7.1 Classical Method of Analysis:
These methods are basically of HISTORICAL interest and are almost never used in practice.
- Method of Consistent distortion or Maxwell-Mohr method,
- Influence Line method by Heinrich Muller-Breslau,
- Three-Moment Theorem,
- Slope Deflection -Displacement Method of Analysis,
 |
| Slope Deflection method of analysis |
7.2 Modern Method of Analysis
A. Approximate Method:
- Portal and Cantilever Method -For Earthquake and Wind Forces,
- ACI Moment Coefficient - For ACI 318-2005-8.9.1,
 |
| ACI Coefficient -Approximate Method |
 |
| Portal Frame- Fig. |
B. EXACT METHOD OF ANALYSIS:
- - Moment Distribution by Hardy Cross.... Link to my Hardy Cross blogpost
- - Matrix Method or Advance method -Using Computer software.
- ETABS software
- PCA Beams software
- MS Spreadsheets software
8. STRESSES Calculations: (NSCP 1.4.2, ACI Code 8.3)
8.1 Bending Moments (Beams, Columns):
8.2 Shear Forces:
8.3 Axial Forces:
8.4 Torsion Forces
References:
- Structural Analysis by Jack C. McCormac, 1997,
- Structural Analysis by R. C. Hibbeler-2012,
- Structural Analysis by Aslam Kassimali -2011,
- Elementary Structural Analysis by C. H. Norris, J.B. Wilbur, S. Utku, 3rd edition-1976,
- Design of Concrete Structures by Arthur H. Nilson, 12th edition -1997,
- Reinforced Concrete Design by Chu-Kia Wang and Charles G. Salmon, 6th edition -1998,
- ACI 318-2002, 2008-Building Code Requirements for Structural Concrete, American Concrete Institute.